Examples of knitting are not something you would expect to find amongst the brass, glass and grey metal boxes that make up a large chunk of the science collections.

Yet, stored away in the National Museums Collection Centre are two pieces of knitted fabric created by the Scottish chemist and mathematician Alexander Crum Brown (1838-1922).

Throughout his career, Crum Brown (or ‘Crummie’ as he was fondly known by the male students who attended his raucous chemistry lectures at Edinburgh University), created models of mathematical or crystal shapes using leather, papier mache, plaster of Paris, and wool to illustrate his research.

He described his models as “helps to the imagination” and James Walker, a fellow chemist and the writer of Crum Brown’s obituary, noted:
“A favourite hobby was the practical construction of tri-dimensional models, both crystallographic and mathematical, a glue pot on the hob and a plentiful supply of cardboard being recognised features of his retiring room in the University.”

As well as making his own models, Crum Brown also collected the work of others. His model of Maxwell’s Thermodynamic Surface, made by James Clerk Maxwell which is on display in Enquire came to us via the Natural Philosophy department of Edinburgh University.
The model illustrating Professor Andrews’ experiments on the relation between the pressure, volume and temperature of carbonic acid is one of three models donated to the museum by Alexander Crum Brown himself.

In his 1861 MD Thesis entitled “On the Theory of Chemical Combination”, Crum Brown was the first to use a graphic representation of organic molecules to show the chemical position of atoms. He represented each atom as a letter inside a circle which were then connected to each other with solid lines. With only small changes, this method of representing molecules is still in use today.
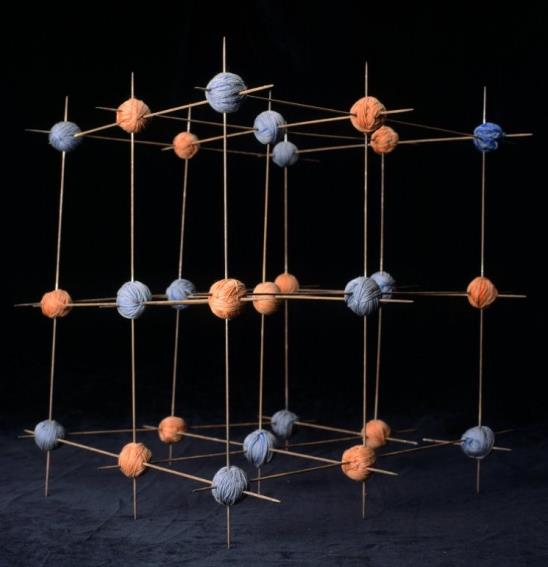
There are examples of Crum Brown’s models in a number of institutions across the UK. This early example of a ‘ball and stick’ model of a rock salt molecule – created using balls of red and blue yarn and steel knitting needles – is in the Chemistry collections at Edinburgh University.

Further examples of his models, demonstrating his work on interlacing and interpenetrating surfaces, can be seen in the collections of the Science Museum.


Science Museum Group Collection © The Board of Trustees of the Science Museum
There are further knitted models are in the Whipple Museum of the History of Science collections. My personal favourite is this set of oak and ivory knitting needles in the care of the Science Museum. I bet they are a dream to work with!

David Dunning, a science historian, came to look at our models a number of years ago. In his subsequent essay, he wrote that Crum Brown’s knitted models not only illustrate his own research on surfaces but also the work of others, in particular, Crum Brown’s colleague and brother-in-law Prof Peter Guthrie Tait (1831-1901).
Tait’s papers on Knot Theory were published by the Transactions of the Royal Society of Edinburgh between 1876 and 1885. The complexity of his research is highlighted In the opening paragraph of ‘On Knots’ (1877) where Tait himself writes:
“The subject is a very much more difficult and intricate one than at first sight one is inclined to think and I feel that I have not succeeded in catching the key note.”
In the essay, Tait attempted to create a classification of knots based on the number of their crossings and their levels of ‘beknottedness’ – basically a description of the complexity of the knot and the number of times that it is necessary to pass one part of the curve of the knot through another in order to untie the knot.
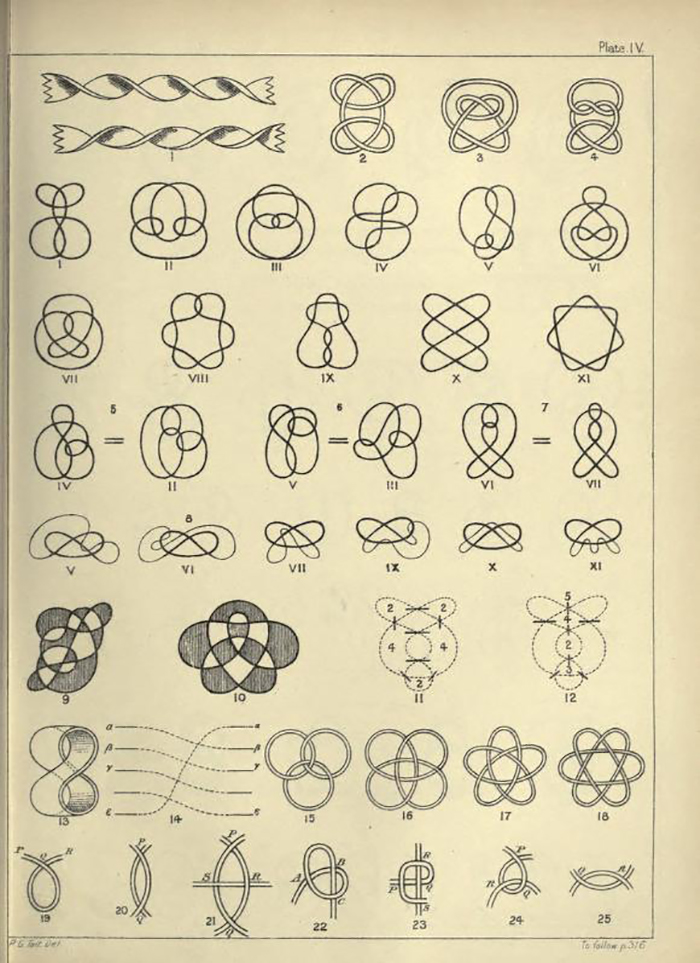
Examples of these various knots are illustrated in Plate IV. If you look at the knot labelled number 16, you’ll see it is identical to the design featured on the largest of our knitted models.

How were the models made?
So we know who made the models and what they were used for but the question that I kept coming back to was how on earth were they made?
As you can see from the photographs, the models consist of three layers of knitted fabric that have been constructed in such a way that the colours appear in a different section of the design in each layer.

When I first looked at the models I thought they were created using a variation of the Double Crochet stitch or were perhaps an example of Tunisian Crochet, but I just couldn’t work out how the three layers of fabric were joined together.
I mentioned the models in a casual conversation with Ruth Churchman – a talented knitter who works at National Museums Scotland and brought them up to Chambers Street for Ruth to inspect and hopefully enlighten me on their construction. Ruth did not disappoint and will now introduce you to the wonderful world of mathematical knitting in part two of this blog.
Read part two of this blog by Ruth Churchman: Alexander Crum Brown’s Mathematical Models: Developing new knitting patterns
